Azchess
It is a variant of Grand Chess. The overall aim of changes are:1. Opportunity of preservation of all game rules from Classical Chess except the castling; 2. To complete the piece set of the game in that all combinations of the moves of the light pieces - Knight, Bishop and Rook (The combination of the specified three figures gives in general four complex pieces). 3. Opportunity of using and inheritance of all theoretical knowledge from a heritage of Classical Chess.
Setup
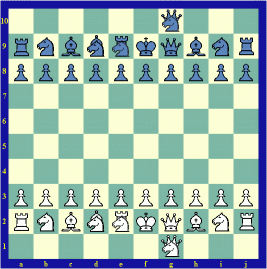
Ten by ten board.Third row (8th row for black) P - Pawns;
Second row from b2 (from b9 for black) R (Rook), N (Knight), B (Bishop), A (Archbishop), C (Chancellor), K (King), Q (Queen), B (Bishop), N (Knight), R (Rook);
First row G (General) - g1.
The Black is symmetrical (a "mirror image") to the White.
Pieces
For the White (the same for the Black) The Pawns - 10; N - Knight (2); B - Bishop (2); R - Rook (2); The Archbishop (A) moves of a Bishop and a Knight; The Chancellor (C) moves of a Rook and a Knight; The Queen (Q) moves of a Rook and a Bishop; The General (G) moves of a Queen and a Knight; The King - White King is located in the dark square and vice-versa, like in Classical Chess.The Cannon (O) - The Cannon moves differently when it moves to capture than when it moves passively. Cannon - moves passively as an Orthodox Rook and moves to capture as an Orthodox Rook which is required to hop over a single screen. In other words, Cannon - capture by hoping over a second piece in order to capture a third piece. For example, Cannon - on a1 can take a piece on f1 when exactly one of the points b1, c1, d1, or e1 is occupied by a piece of either color. Cannon - only capture when hoping and only hop when capturing. They may never hop over more than one piece in a given move. Cannon needs an intermediate piece between itself and its victim. The Cannon jumps the intermediate and take the victim on its square. The intermediate is left unaffected.
G = Q + N = R + B + N, it is a very powerful piece, capable of giving mate by itself.
Q = R + B
C = R + N
A = B + N ; For a large board B > N; R > O > B;
Rules
The game follows the standard Classical Chess rules without exceptions and the Pawns may be promoted to Archbishop, Chancellor and General also. The castling is impossible.Figure 1. General view (Mirror symmetry)
Notes
The General(G) is located on the square g1, because in this case all Pawns are protected very well. From a3 to j3 like this:1 2 2 2 3 4 5 2 1 1.
The arrangement of the strongest figure(G) on last horizontal as though symbolizes the commander-in-chief supervising the chess army in deep rear. With an opportunity of fast movement from flank on flank. As many powerful pieces participate in game and question of vulnerability of the weakest piece (K) is actual. Therefore offered setup of pieces is complete. In the King side total of protection of Pawns equally 13 and in opposite flank it is 10. Presence of a free horizontal in rear of the King raises resources of defense, that enables retreat of the King at powerful attacks by the adversary.
The name of this game may be "Azchess". I used first and last letter of the English alphabet for this name, because in my opinion it would symbolize infinity of a Chess game. The game well balanced. Two diagonal power lines (Bishop and Archbishop) symmetric to another two diagonal power lines (Queen and Bishop). All straight power lines are well directed on flanks (Rooks) and to the central squares (Chancellor and Queen). Azchess has following advantages above Classical Chess.
1. The quantity of various types of positions increases, for example: for Classical Chess maximum quantity of positions is 13 in a degree 64; for Azchess - 19 in a degree 100. 2. The large board (10x10) and new pieces (A, C, G) allow to receive new and interesting chess endings, for example: A vs C; G vs Q and so on. 3. Presence of last empty horizontal line (the line 1 for White; the line 10 for Black) increases resources of defense, 4. In Classical Chess a King has "one line of pawns" for defense, but in Azhess a King has "extra line of pieces" for defense, too.
If to look more closely to opening setup of many Chess games, it is possible to notice undistinguished but exclusively important distinction. Concerning an average horizontal line the Black pieces are "mirror image" to the white pieces (a line symmetry). The "mirror image" does left by right, upwards by a bottom and so on. For this reason, White King is located on a dark square against Black King which is located on a light square. Thus a Black Army automatically become "a mirror double" to a White Army. It is impossible to put an equal-sign between them. They are strongly differ by the nature. The central symmetry stand above in comparison with linear symmetry and also is more aesthetic. For the maximal equalizing of a game, and reduction of advantage of the first move to a minimum a "mirror or line symmetry" of opening setup must be changed to a "revolving symmetry" or a "central symmetry".
Figure 2. General view (Central symmetry)

A figure is called centrally symmetric with respect to a single point if, on a reversal with respect to it, it covers itself .
For both Army pawns protection:
Black: 1 1 2 5 4 3 2 2 2 1
White: 1 2 2 2 3 4 5 2 1 1
The Basic condition of Chess games: "contenders should have equal opportunities before game", it is the basic axiom. The start position for both Army is absolutely symmetrical from the point of view of mathematics. All double pieces are located on the squares of same color. The midpoint of the revolving symmetry is located between squares e5, f5 and e6, f6.
In Figure 3 are shown Azchess with extra Cannons.
Figure 3, Basic Setup

 This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
By Namik Zade.
Last revised by H. G. Muller.
Web page created: 2006-03-19. Web page last updated: 2022-11-25