Comments/Ratings for a Single Item
I don't usually like games with different armies, but this is an exception. You've put a lot of thought into making a game whose different armies are not unevenly matched. For sure, the Spartan side lacks a Queen and its army appears to be slightly less powerful, but that is compensated for by the presence of two kings, both of which must be checkmated/captured.
Heck yeah!
Then let's play! It looks like a CWA theme game.
 Greg Strong wrote on Fri, Mar 31, 2017 03:58 PM UTC:
Greg Strong wrote on Fri, Mar 31, 2017 03:58 PM UTC:I think people have always felt free to implement presets that had not yet been created. It is unlikely there would be an objection.
In the case of Spartan Chess, I know the inventor wanted a GC preset and had created some new piece graphics to be added to the Alfaerie set for that purpose. This was probably about 6 years ago, but they should be in my email. I will dig them up and get them uploaded and get a Piece Set created so that the pieces can be identified by proper notation.
I see a lot of work has been put into this game and it has been favorited by several people. However, I don't see a Game Courier preset. Is there one of these currently and if not, would it be violating any copyright policies to impliment one? Seems odd to have one of the top favorited games on the site without a preset.
 H. G. Muller wrote on Thu, Oct 20, 2016 02:22 PM UTC:Excellent ★★★★★
H. G. Muller wrote on Thu, Oct 20, 2016 02:22 PM UTC:Excellent ★★★★★|
holdingsType=1
promoZone=1
maxPromote=2
promoChoice=*N*B*R*Q*C*L*G*W*K
graphicsDir=http://www.chessvariants.com/membergraphics/MSelven-chess/
whitePrefix=w
blackPrefix=b
graphicsType=png
startShade=#FFCC00
symmetry=none
pawn::fmWfcFifmnD::a2-h2,,
hoplit::fmFfcWifmA:lance:,,a7-h7
knight:N:::b1,g1,,:8,0
bishop:B:B::c1,f1,,:8,0
rook::::a1,h1,,:8,0
queen::::d1,,:8,0
captain::WD:tower:,,d8,e8:0,8
lieutenant::FAsmW:elephant:,,a8,h8:0,8
general::RF:crownedrook:,,b8:0,8
warlord::BN:archbishop:,,g8:0,8
king (spartan)::K:king:,,c8,f8
king (persian)::KisO2:king:e1,,
Color coding of moves:
|
Spartan ChessThis game is one of the top favorites of this site. As it also does feature a fair number of unorthodox pieces that makes it deservant of an interactive diagram. Especially since almost all comments on it have been totally garbled by messing up their layout. |
Because of the asymmetry of this variant it required some special attention to make sure the diagram would only allow each side to promote to its own pieces. What I did was define the promotion choice with an asterisk before each piece, meaning it can only come from the holdings. Then I stuffed the holdings initially with eight of each eligible piece type (enough to promote all Pawns to it).
Except for the Spartan King, which would only be a valid promotion choice if one of the Kings was captured. So I defined the holdings type such that it would accumulate captured pieces of the original owner. By starting without Kings in hand the Spartans then can only promote to King when one of their Kings is captured.
Castling
Only the Persians can castle, and this forced the Persian and Spartan Kings to be defined as different piece types. (If castling is specified with the aid of an O atom, the diagram assumes it is with a Rook or a corner piece of any type. And we do'nt want the Spartan Kings to castle with their Lieutenants!) This has the dubious effect of the Spartan Kings not being considered royal (as the diagram only considers one piece royal, by default the last one defined). So moves of Spartan Kings into check are not 'grayed out', like they are for the Persian King. But of course this is justifiable, as the Spartan King can actually expose itself to capture (if there still is a second one). So such moves can be legal. The diagram does not understand extinction royalty.
An approximate setup using the alfaerie pieces: /play/pbm/play.php?game=Anti-King%2520Chess%2520II&set=alfaerie-many&flip=1&movelist=1.%2520k%2520e8-f8%2520%250A1...%2520k-c8%2520%250A2.%2520.rf-b8%2520%250A2...%2520x-h7%253B%2520x-g7%253B%2520x-f7%253B%2520x-e7%253B%2520x-d7%253B%2520x-c7%253B%2520x-b7%253B%2520x-a7%2520%250A3.%2520.dw-d8%253B%2520.dw-e8%2520%250A3...%2520.ef-a8%2520%250A4.%2520.ef-h8&movenum=7&seed=1957544903&game=Anti-King%2520Chess%2520II&turn=4&moves=a-g8&submit=Move&_render=table&_shape=square&_scale=100&_set=alfaerie-many&selectcolors=&_colors=339933+CCCC11+22BB22&_bcolor=111199
I'd suggest changing the "punchline" to something more descriptive than "http://www.spartanchessonline.com". Suggestion: "The spartan army with 2 Kings and novel pieces fights against the persians (standard chess army)" The punchline occurs in several listings on this site, including the favourite games listing.
A Spartan chess set is now designed and prototyped.
The prototypes set is available for sale on Shapeways: Spartan Set  Â

Spartan Chess Puzzles - Solutions Puzzle #1 Black (Spartan) to move and mate in 3 1. ... Lf3-e3+ 2. Kb1 Cb5-b3+ 3. Ka1 L35-c3# ---------------------------------------------- Puzzle #2 Black (Spartan) to move and mate in 4 1. ... Hb2-c1 = K 2. Qa2xKc2 Kc1xQc2 3. Ka1-a2 Ca5-a4+ 4. Ka2-a1 Ca4-a3# Only by promotion the hoplite to a King can the Spartan win. Persian moves other than 2. Qa2xKc2 result in a quicker Spartan victory. 2. Qa2-b3 Ca5-a3+ 3. Qb3xCa3 Kc1-b1# 2. (any other) Kc1-b1# ---------------------------------------------- Puzzle #3 White (Persian) to move and mate in 2 1. Nd8-b7+ Ld5xNb7 2. b2-b4# ---------------------------------------------- Puzzle #4 Black (Spartan) to move and mate in 3 1. ... Lf5-c3+ 2. Bb2xLc3 La5xBc3+ 3. Rf3xLc3 Wb4xRc3# ---------------------------------------------- Puzzle #5 Black (Spartan) to move and mate in 2 1. ... Wc5xb3+ 2. Kc1-b2 Hd2-c1=W#
The solutions will be posted separately in a day or so.
Spartan pieces are labeled and move as follows:
h = Hoplite - moves 1 square diagonally and captures 1 forward
L = Lieutenant - leaps 1 or 2 squares diagonally, moves (no capture) 1 square horizontally
C = Captain - leaps 1 or 2 squares orthogonally
G = General - moves like a rook or King
W = Warlord - moves like a Knight or a Bishop
K = Spartan King - move exactly like an orthodox King
Puzzle #4
Black (Spartan) to move and mate in 3
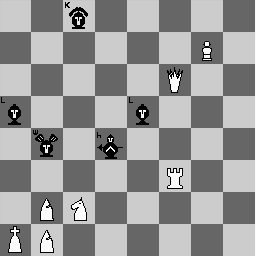
Puzzle #5
Black (Spartan) to move and mate in 2

Mr. Anonymous
----------------------
puzzle 2 a5-a3+ a2-a3
b2-b1+ promotes
if promote to RN, then a1-a2
b1-c3+ a2-a1
c3-a3 mate
if promote to RK [aka: General], then mate.
----------------------
b2-b1 is not a correct Spartan Chess move. The hoplite, or Spartan pawn, moves diagonally and captures straight ahead. Since there is no enemy piece on b1 the hoplite cannot get to that square. Also there is no RN. There is the General=RK and the Warlord=BN.
The solution is a bit trickier.puzzle 2 a5-a3+ a2-a3
b2-b1+ promotes
if promote to RN, then a1-a2
b1-c3+ a2-a1
c3-a3 mate
if promote to RK [aka: General], then mate.puzzle 1 f3-e3+ c1-b1
b5-b3+ b1-a1
e5-c3 mateShortly I will post their solutions or the refutation as it is easy to err on such puzzles.
Spartan pieces are labeled and move as follows:
h = Hoplite - moves 1 square diagonally and captures 1 forward
L = Lieutenant - leaps 1 or 2 squares diagonally, moves (no capture) 1 square horizontally
C = Captain - leaps 1 or 2 squares orthogonally
G = General - moves like a rook or King
W = Warlord - moves like a Knight or a Bishop
K = Spartan King - move exactly like an orthodox King
Puzzle #1
Black (Spartan) to move and mate in 3

Puzzle #2
Black (Spartan) to move and mate in 4

Puzzle #3
White (Persian) to move and mate in 2

Post your solution, comment or critique.
If you create a Spartan chess puzzle please post it and I will be glad to
post it to the official Spartan Chess website crediting you as its author.
I have used & nbsp (with no space between & and n) as non-breaking space several times on this site. One can also use & #151 which is long dash (with no space between & and n), etc. Then it looks like this:  and this: — /Mats
Gah! I see what you mean. They were not like this at one point, now they are. Thanks for pointing it out. I will get right on it. Hmmm, sort of odd. I resubmitted the first section and all the offending characters were gone. When I resubmitted the second section, The Setup, the offending characters were back. It seems that what is not liked is '& nbsp;' (no space between & and nbsp;) which is used in HTML for padding spaces. I will figure a way to scrub this out of the submission. Scrubbing done. Still seems sort of odd. Is there a character or string of characters we use on this site for a non-breaking space?
 H. G. Muller wrote on Tue, Feb 15, 2011 09:51 PM UTC:
H. G. Muller wrote on Tue, Feb 15, 2011 09:51 PM UTC:Very nice. It shows the power of a jump. A diagonal slider can seldomly do this (except in fool's mate), but here it does not matter that d2 is defended several times. Blocking the check is not possible. I guess tactical motifs like this are what makes a pair of Lieutenants about equivalent to a Bishop pair. Something that much surprised me. Apparently the single non-blockable 2-square jump has nearly the same value as all distant moves of a slider, which can be blocked.
1. c4 ... Hac5
2. b3 ... Hxc4
3. bxc4 ... Hc5
4. d3 ... Lf6
5. Na3 ... Le5
6. Nf3 ... Lc3#
Final Position:

By the way...
I consider c4, the first move played by the Persian in this losing game,
to be the strongest first move and consider e4, the most common in
orthodox chess, to be among the weakest. Some analysis to follow.
 H. G. Muller wrote on Sat, Feb 12, 2011 11:56 AM UTC:
H. G. Muller wrote on Sat, Feb 12, 2011 11:56 AM UTC:I did run some 5-men Spartan end-games with my tablebase generator, and can confirm that the following endgames are all 'generally won' to white:
moves to conversion:
max typ
K+Q+B vs K+W 44 10
K+Q+N vs K+W 45 10
K+Q+B vs K+G 33 18
K+Q+N vs K+G 39 18
'Generally won' means that with white to move it is virtually always won, except for the ~1% of cases where the initial position happens to be a fork or skewer against your K+Q. With black to move these are only won in about 35% of the cases, because in the remaining 65% the powerful black defender (W or G) will be able to capture either King or Queen in the initial position.
The first two end-games can already be found on this site (calculate by McCooey, where Warlord is called Pegasus), those with the General are new.25 comments displayed
Permalink to the exact comments currently displayed.

Spartan Chess has the Warlord and the General, together worth approximately three Rooks. These are solid performers that have been used in many variants. Worth noting that pieces like the Lieutenant with additional noncapturing moves are rare. My Shatranj Kamil (64) has Elephants with noncapturing Dabbabah leaps and ArchMage Chess by Cyrus Arturas has the Prince, a Commoner with noncapturing R2 moves (no mention of Dabbabah leaps). In the diagram below, a Warlord pins a Rook against the White King - winning the piece and the game.
WHITE TO MOVE AND LOSE